- Introduction
- TL;DR
- Understanding the Basics of Time Constants
- The Time Constant Formula
- Time Constants in RC and RL Circuits
- Practical Applications of Time Constants
- Misconceptions About Time Constants
- Why Choose Keysight for Your Electrical Engineering Needs
- Glossary of Terms
- Final Thoughts from the Keysight Used Store
- Whenever You’re Ready, Here Are 5 Ways We Can Help You
- Find Used Oscilloscopes On Sale
"In the world of electrical engineering, time constants are not just numbers; they are the heartbeat of circuitry. Mastering their calculation is a ticket to the control of every oscillation and transient response." – Dr. James K. Roberge, Former Professor of Electrical Engineering, MIT.
It's hard to overstate the importance of understanding time constants in electrical engineering. Whether it's the rapid pulse of a high-frequency oscillator, the precise tuning of a radio station, or the intentional delay in a motor start-up, time constants are at the core. They are essential for providing a deep understanding of how circuits respond over time.
One could argue that the life of an electrical engineer is spent in the careful orchestration of time. Consider, for example, designing a system to control the power flow in an electric vehicle. The challenge isn't just about ensuring enough power to run the vehicle, but also about carefully managing how quickly power levels rise and fall, avoiding any sudden changes that might harm the vehicle's delicate electronics. In this context, the time constant—the rate at which a circuit rises or falls in response to a change—becomes a critical variable that engineers need to understand.
We'll be exploring the art of calculating time constants in RC (Resistor-Capacitor) and RL (Resistor-Inductor) circuits, as it is not merely a process of plugging values into a formula, but a skill that can profoundly affect the behavior and performance of an electrical system. Once mastered, this seemingly abstract concept will become a tangible tool, enabling you to shape the flow of current and voltage in a way that can make or break your design.
TL;DR
- Time constant, denoted as 'τ', is a crucial concept in electrical engineering, measuring the response time of a system to a step input. In an RC circuit, τ = RC, and in an RL circuit, τ = L/R.
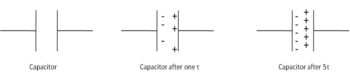
- After one time constant, an RC or RL circuit reaches approximately 63.2% of its final value, not 100%. The system is generally considered to have reached its final value after about 5 time constants.
- The time constant of a circuit may vary due to changes in resistance, capacitance, or inductance, which may depend on factors like temperature.
- Time constants have a variety of applications in electrical devices, from shaping signals in oscilloscopes to timing the flash in a camera.
- Keysight provides high-quality, thoroughly tested and certified refurbished equipment, offering excellent support to electrical engineers. Our used equipment store offers access to a range of devices, from oscilloscopes to signal generators, backed with comprehensive calibration services, warranty, and technical support.
- Understanding and correctly applying time constant knowledge can significantly enhance the efficiency and reliability of work in electrical engineering.
Understanding the Basics of Time Constants
In the realm of electronics, a time constant, denoted by the Greek letter 'τ' (tau), measures the speed at which a system responds to changes. Specifically, it's the time required for a system's response to reach approximately 63.2% of its final value following a step input.
For an RC (Resistor-Capacitor) circuit, the time constant τ is calculated by multiplying the resistance (R, in ohms) by the capacitance (C, in farads), giving us a time constant in seconds: τ = RC
For an RL (Resistor-Inductor) circuit, the time constant is the inductance (L, in henrys) divided by the resistance (R, in ohms), again yielding a time constant in seconds: τ = L/R
In both cases, the time constant gives an indication of how quickly the circuit responds to changes in input. A small time constant implies a quick response, while a larger time constant indicates a slower response.
In electrical engineering, time constants are essential because they dictate the behavior of circuits in the time domain. They determine how quickly capacitors charge and discharge in filters, timing circuits, and power supplies, or how swiftly current builds and diminishes in inductors found in transformers, motors, and transmission lines.
Thus, understanding and accurately calculating time constants is crucial in designing and optimizing circuits for desired performance.
| Key Takeaway |
|---|
| In electrical engineering, "time constant" is a basic concept represented by the symbol 'τ'. It measures how quickly a system responds to a step input. In RC and RL circuits, the time constant can be calculated using the formulas τ = RC and τ = L/R respectively, and it plays a crucial role in determining the behavior and performance of circuits. |
The Time Constant Formula
The time constant formulas for RC (Resistor-Capacitor) and RL (Resistor-Inductor) circuits are mathematical representations of the circuits' transient behavior—how the current and voltage change over time.
For an RC circuit, the time constant τ is determined by the product of resistance (R) and capacitance (C):
τ = RC
For an RL circuit, the time constant τ is calculated by dividing the inductance (L) by the resistance (R):
τ = L/R
Let's delve into the components of these formulas and their significance:
| Component | Symbol | Units | Significance |
| Resistance | R | Ohm (Ω) | Determines how much the circuit resists the flow of current. A higher resistance leads to a longer time constant, slowing the circuit's response. |
| Capacitance | C | Farad (F) | In an RC circuit, it quantifies the amount of energy the capacitor can store. A higher capacitance leads to a longer time constant, slowing the charge and discharge rate. |
| Inductance | L | Henry (H) | In an RL circuit, it measures the amount of energy the inductor can store in its magnetic field. It is a measure of how much a conductor opposes a change in current. A higher inductance leads to a longer time constant, slowing the rise and fall of current. |
Key factors that affect the time constant in RC and RL circuits include:
- Resistance (R): In RC systems, a higher resistance increases the time constant, slowing the response. In RL systems, a higher resistance leads to a decrease in time constant
- Capacitance (C): In RC circuits, a higher capacitance increases the time constant, slowing the charging and discharging rate of the capacitor.
- Inductance (L): In RL circuits, a higher inductance increases the time constant, slowing the rate at which current changes.
Understanding these factors allows engineers to manipulate the time constants to suit the needs of their designs. For example, in a power supply filter, a longer time constant (achieved with different or higher resistance, inductance or capacitance) could help smooth out voltage variations more effectively. Conversely, in a radio tuning circuit, a shorter time constant might be preferred for a quicker response to frequency changes.
Time Constants in RC and RL Circuits
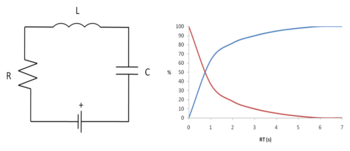
In RC and RL circuits, the time constant is a measure of how long it takes for the voltage or current to rise or fall.
In an RC circuit, when a voltage is suddenly applied (step input), the capacitor gradually charges up through the resistor, reaching about 63.2% of the applied voltage after one time constant. It will continue to charge until it reaches near 100% of the applied voltage, typically considered fully charged after about 5 time constants (or 5τ).
Conversely, if the voltage source is suddenly removed, the capacitor discharges through the resistor. The voltage across the capacitor decreases over time, dropping to about 36.8% of its original voltage after one time constant. After approximately 5 time constants, the capacitor is considered fully discharged.
In an RL circuit, when a voltage is suddenly applied, the current through the circuit does not immediately reach its maximum value. Instead, it slowly increases and reaches about 63.2% of its maximum value after one time constant. After about 5 time constants, the current is essentially at its maximum value.
If the voltage source is suddenly removed, the current starts to decrease, dropping to about 36.8% of its original value after one time constant. After about 5 time constants, the current is considered to have reached zero.
Steps to calculate time constants in RC and RL circuits:
- Identify the resistance (R) and either the capacitance (C) for RC circuits or inductance (L) for RL circuits. These values are often given in circuit diagrams or can be calculated based on the components used.
- Use the time constant formulas τ = RC for RC circuits and τ = L/R for RL circuits.
- Plug in the values for R, C, or L, ensuring they are in the correct units (ohms for R, farads for C, henrys for L).
- Calculate the time constant, which will be in seconds.
| Key Takeaway |
|---|
| Understanding time constants in RC and RL circuits is crucial for designing efficient electronic circuits. This concept helps in predicting the transient behavior of circuits, which in turn influences aspects like filter performance, timing operations, and system stability. By mastering the calculation of time constants, electrical engineers can manipulate circuit responses to meet specific design requirements. |
Practical Applications of Time Constants
Time constants play an integral role in the design and functioning of many electronic devices and circuits. They determine the rate at which energy is stored or released, affecting the performance of filters, the timing in digital circuits, the speed control in motors, and even the frequency tuning in communication devices.
For example, in a simple electronic flash for a camera, an RC circuit is used. The charging time of the flash (how long it takes the flash to be ready after a picture is taken) is determined by the RC time constant of the circuit.
In a radio, RL and RC circuits are used for tuning to select the desired frequency. The time constant of these circuits affects the sharpness of the tuning (how well the radio separates different stations).
Oscilloscopes and signal generators also heavily depend on time constants. In oscilloscopes, RC circuits help to shape the signals for accurate time measurements. Similarly, signal generators often use RL or RC circuits to produce different types of waveforms, with the time constant controlling the frequency and shape of the output signal.

| Application | Circuit Type | Role of Time Constant |
| Electronic Flash | RC | Determines the charging time of the flash |
| Radio Tuning | RL/RC | Affects the sharpness of frequency tuning |
| Oscilloscopes | RC | Helps in accurate time measurement of signals |
| Signal Generators | RL/RC | Controls the frequency and shape of output signal |
Key benefits of understanding time constants in electrical engineering include:
- Predicting Circuit Behavior: Knowing the time constant can help predict how a circuit will react to changes in input, which is crucial in design and troubleshooting.
- Optimizing Performance: By manipulating the time constant, engineers can optimize a circuit's performance, such as improving the response speed or stability.
- Versatile Design: Knowledge of time constants allows engineers to create circuits that perform a wide range of functions, from filtering signals to timing operations.
- Improved Safety: Understanding time constants can help engineers design circuits with safer responses to sudden changes, such as power surges.
Time constants bridge the gap between theory and practice in electrical engineering, turning abstract mathematical formulas into tangible tools for creating efficient and effective electronic devices.
Misconceptions About Time Constants
While time constants are fundamental to electrical engineering, misconceptions about them can lead to confusion or inaccuracies. Let's debunk some of the most common misconceptions:
- Misconception: After one time constant, the system reaches its final value.
Correction: In reality, after one time constant, an RC or RL circuit reaches approximately 63.2% of its final value, not 100%. The system is generally considered to reach its final value after about 5 time constants (5τ). - Misconception: The time constant is always fixed for a particular circuit.
Correction: The time constant is determined by the values of resistance, capacitance, or inductance in a circuit, which can be variable. For example, in a temperature-dependent circuit, resistance may change with temperature, thus altering the time constant. - Misconception: Time constants only apply to first-order systems (like simple RL or RC circuits)
Correction: While time constants are most straightforward in first-order systems, they can also apply to higher-order systems, such as circuits with multiple resistors, capacitors, or inductors. These more complex systems may have multiple time constants, each related to different aspects of the system's behavior.
| Key Takeaway |
|---|
| Misconceptions about time constants can lead to misunderstandings of electrical circuit behavior. However, by seeking accurate information and applying it effectively, you can enhance your proficiency in electrical engineering and improve your circuit designs. |
Why Choose Keysight for Your Electrical Engineering Needs
Keysight Technologies is a leading provider of electronic design and test solutions, offering a range of state-of-the-art equipment, from oscilloscopes to signal generators. Opting for Keysight's certified refurbished equipment can be a cost-effective choice that delivers performance, reliability, and customer satisfaction.
One of the key benefits of buying used equipment from Keysight is that all refurbished devices go through a comprehensive refurbishment and testing process. This ensures that the equipment meets the same functional standards as new products.
Keysight also offers unique calibration services. Their calibration labs are certified and fully equipped with top-tier metrology equipment. Keysight's calibration services ensure that your equipment continues to meet its original specifications, maximizing performance and lifespan.

Key benefits of choosing Keysight-certified refurbished equipment for your electrical engineering needs include:
- Reliability: All Keysight refurbished products undergo a thorough refurbishment and testing process, ensuring they meet the same functional performance as new products.
- Savings: Keysight's refurbished equipment offers an excellent way to obtain high-quality test and measurement equipment at a reduced cost.
- Comprehensive Calibration Services: Keysight offers industry-leading calibration services to ensure your equipment continues to perform to its original specifications.
- Support and Warranty: Keysight's refurbished products come with a warranty and have the backing of Keysight's technical support team, offering peace of mind to customers.
Whether you're outfitting a new lab or extending your test and measurement capabilities, Keysight's certified refurbished equipment offers a combination of quality, reliability, and value that's hard to beat.
Glossary of Terms
Understanding the terminology associated with time constants can enhance comprehension and enable more effective communication in the field of electrical engineering. Below are some key terms:
| Term | Definition | Example |
| Time Constant | The time required for a system's response to reach approximately 63.2% of its final value following a step input. | In an RC circuit, the time constant τ = RC. |
| Resistance (R) | The opposition that a substance offers to the flow of electric current. Measured in Ohms (Ω). | A resistor in a circuit might have a resistance of 10 Ω. |
| Capacitance (C) | The ability of a component to store an electric charge. Measured in Farads (F). | A capacitor in a circuit might have a capacitance of 100 μF (microfarads). |
| Inductance (L) | The property of an electrical conductor by which a change in current flowing through it induces an electromotive force in the conductor itself and in any nearby conductors. Measured in Henrys (H). | An inductor might have an inductance of 200 mH (millihenries). |
| Step Input | A sudden change in the input signal. | Applying a voltage to a previously unpowered circuit. |
| Transient Behavior | How a circuit responds over time to a change in the input. | The charging of a capacitor or the current rise in an inductor are examples of transient behavior. |
| Key Takeaway |
|---|
| A solid grasp of the key terms and definitions related to time constants can facilitate a deeper understanding of the concept, aiding in both practical applications and theoretical discussions. With this lexicon, you can effectively describe and analyze the transient behavior of electrical circuits. |

Final Thoughts from the Keysight Used Store
Understanding time constants is crucial for anyone involved in the design or analysis of electronic circuits. Whether you're working with an RC circuit where the time constant τ = RC or an RL circuit where τ = L/R, this concept is key in predicting the transient behavior of these systems. Familiarity with these formulas, and the ability to apply them effectively, can significantly enhance your work's efficiency and reliability.
Time constants offer a way to calculate how quickly circuits respond to changes, influencing aspects like filter performance, timing operations, and system stability. Misconceptions about time constants can lead to inaccuracies, but with the correct understanding, electrical engineers can manipulate circuit responses to meet specific design requirements.
At Keysight, we're committed to supporting engineers in applying these crucial principles. Through our used equipment store, we provide access to high-quality, thoroughly tested, and certified refurbished equipment, ensuring that engineers can apply their time constant knowledge effectively. Our range of devices, from oscilloscopes to signal generators, backed with comprehensive calibration services, warranty, and technical support, can help engineers bring their projects to life with confidence and efficiency.
We encourage you to explore Keysight's used store for further resources and tools tailored for electrical engineers. Here, you can find not only equipment but also educational resources that can deepen your understanding of essential electrical engineering concepts like time constants.
Mastering the art of calculating time constants is a valuable skill in electrical engineering, bridging the gap between theory and practice, and paving the way for effective and efficient circuit design and analysis.

Whenever You’re Ready, Here Are 5 Ways We Can Help You
- Browse our Premium Used Oscilloscopes.
- Call tech support US: 1 800 829-4444
Press #, then 2. Hours: 7am – 5pm MT, Mon– Fri - Talk to our sales support team by clicking the icon (bottom right corner) on every offer page
- Create an account to get price alerts and access to exclusive waitlists
- Talk to your account manager about your specific needs
