- Introduction
- What is Potential Energy?
- Discover Quality Premium Used Equipment for Precision Measurements
- Types of Potential Energy
- Gravitational Potential Energy
- Elastic Potential Energy
- Electrical Potential Energy
- Derivation of Potential Energy Formulas
- Gravitational Potential Energy
- Elastic Potential Energy
- Electrical Potential Energy
- How to Calculate Potential Energy
- Gravitational Potential Energy
- Elastic Potential Energy
- Electrical Potential Energy
- Potential Energy vs Kinetic Energy
- Kinetic Energy
- Relationship Between Potential and Kinetic Energy
- Comparison Table
- Real-World Examples of Potential Energy Calculations
- Example 1: Potential Energy of a Book on a Shelf
- Example 2: Potential Energy of a Roller Coaster at the Top of a Hill
- Example 3: Potential Energy of Water Stored in a Dam
- Practical Applications in Engineering and Technology
- Tools for Measuring Potential Energy
- Oscilloscopes
- Other Relevant Tools
- Practical Tips for Tool Selection and Use
- Comparison Table
- Choosing Premium Used Equipment
- Ensure Accuracy with Keysight's Calibration Services
- Whenever You’re Ready, Here Are 5 Ways We Can Help You
Picture this: you’re designing a capacitor bank for an energy storage system. The system is meant to store electrical energy when the supply exceeds demand and release it during peak usage.
To ensure the system functions reliably, you need to understand how potential energy is stored in the electric field between the capacitor plates. If your calculations are off, the stored energy could either fall short or, worse, cause overheating and damage to the system.
Knowing how to accurately measure potential energy in an electrical system is essential for balancing performance and safety. By understanding the core concepts behind potential energy, you'll be better equipped to design systems that operate efficiently and avoid costly mistakes.
What is Potential Energy?
Potential energy is the energy an object stores due to its position or configuration. The most common form is gravitational potential energy, which depends on the object's mass, height, and the gravitational force acting on it. Mathematically, this form of potential energy is expressed as:
U=m×g×h
U = potential energy \((m^2/s^2 or J)\)
m = mass (g)
g = gravitational acceleration \((m/s^2)\)
h = height (m)
Potential energy plays a key role in understanding how energy is conserved and transferred within physical systems. By knowing the factors influencing potential energy, you can predict how much work an object can do when its stored energy is released. This insight is crucial in fields ranging from mechanical design to energy efficiency analysis.
Discover Quality Premium Used Equipment for Precision Measurements
Types of Potential Energy
Potential energy comes in several forms, each associated with the position or configuration of an object within a force field, such as gravity, elastic tension, or an electric field.
Understanding these types of potential energy is essential for engineers, as they are fundamental to predicting how energy will be stored and transferred within a system.
The three main types of potential energy—gravitational, elastic, and electrical—are the most commonly encountered in various fields of engineering and physics.
Gravitational Potential Energy
Gravitational potential energy is the energy stored in an object due to its position in a gravitational field. When you lift an object above the ground, you are doing work against gravity, storing energy in the object that can be released when it falls. The formula for calculating gravitational potential energy is:
U = m × g × h
Where:
- m = mass of the object
- g = gravitational acceleration (9.8 m/s² on Earth)
- h = height above the reference point
Elastic Potential Energy
Elastic potential energy is the energy stored in objects that can be stretched or compressed, such as springs, rubber bands, or bungee cords. This energy is stored when a force is applied to deform the object and released when the object returns to its original shape.
Hooke's Law describes the relationship between the force applied to stretch or compress an object and the resulting deformation:
F = -k × x
Where:
- F = force applied
- k = spring constant (a measure of the stiffness of the spring)
- x = displacement from the equilibrium position
Electrical Potential Energy
Electrical potential energy is the energy stored in an object due to its position in an electric field. This type of energy occurs when charged particles, like electrons or protons, are subjected to electric forces.
Unlike gravitational and elastic potential energy, electrical potential energy deals with the forces between electric charges rather than mass or deformation.
The electrical potential energy between two charges can be expressed as:
\(U=-k_e \frac{q_1q_2}{r}\), where
- Ke = Coulomb constant
- q = charges
- r = separation
Derivation of Potential Energy Formulas
The mathematical derivation of potential energy formulas stems from the work-energy principle, which states that the work done on an object is equal to the change in its energy. For potential energy, this principle helps us understand how energy is stored within a system due to the position or configuration of objects. Below, we’ll derive the formulas for gravitational, elastic, and electrical potential energy using basic principles.
Gravitational Potential Energy
The derivation of the gravitational potential energy formula begins with the concept of work. Work is defined as the force applied to an object times the displacement over which the force is applied:
Work (W) = Force (F) × Displacement (d) × cos(θ)
In the case of gravitational potential energy, the force acting on the object is its weight, which is given by the object's mass (m) multiplied by gravitational acceleration (g):
Force (F) = m × g
When the object is raised vertically, the displacement is equal to the change in height (h), and the angle θ between the force of gravity and the displacement is 0° (cos(0°) = 1), so the work done on the object becomes:
W = m × g × h
Since the work done in lifting the object is stored as gravitational potential energy (U), we get the gravitational potential energy formula:
U = m × g × h
Assumptions and Approximations:
- The gravitational acceleration (g) is constant and uniform (9.8 m/s² near the Earth's surface).
- The reference point (h = 0) is arbitrary and chosen based on the system under consideration.
Elastic Potential Energy
The derivation of elastic potential energy relies on Hooke's Law, which states that the force needed to stretch or compress a spring is proportional to the displacement (x) from its equilibrium position:
Force (F) = -k × x
Where k is the spring constant, representing the stiffness of the spring. The negative sign indicates that the force opposes the displacement. The work done in stretching or compressing the spring is calculated by integrating the force over the displacement:
\(W=\int Fdx= \int(-kx)dx\)
The integral of this expression from 0 to x (where x is the displacement) gives:
W = 1/2 × k × x²
This work done is stored as elastic potential energy (U) in the spring, leading to the formula:
U = 1/2 × k × x²
Assumptions and Approximations:
- The spring follows Hooke's Law perfectly (linear relationship between force and displacement).
- Deformations remain within the elastic limit, meaning the spring returns to its original shape after the force is removed.
Electrical Potential Energy
The electrical potential energy between two point charges arises from the work done in moving one charge in the electric field created by the other. Coulomb's Law provides the force between two point charges (q₁ and q₂) separated by a distance (r):
Force (F) = ke × (q₁ × q₂) / r²
Where \(k_e\) is Coulomb's constant. The work done to move a charge against this force is the integral of the force with respect to distance:
\(W= \int Fdr= \int k_e \frac{q_1q_2}{r^2} dr\)
The integral of this expression from \(r_₀\) to r gives:
\(W= k_e \frac{q_1q_2}{r} \)
This work is stored as electrical potential energy (PE), so the formula becomes:
\(U= k_e \frac{q_1q_2}{r}\)
Assumptions and Approximations:
- The charges are point charges, meaning they are treated as if all their charge is concentrated at a single point.
- The medium between the charges is a vacuum or air, so the permittivity of the medium is considered constant.
The derivation of potential energy formulas is rooted in the work-energy principle, which helps us understand how energy is stored in systems based on forces and displacements.
By following step-by-step derivations for gravitational, elastic, and electrical potential energy, we can better appreciate the mathematical foundation behind these key concepts.
Assumptions and approximations in the derivations simplify complex real-world scenarios, allowing engineers to model and calculate potential energy accurately in various applications.
How to Calculate Potential Energy
Calculating potential energy is an essential part of understanding how energy is stored within a system. Depending on the system you're working with—whether it’s gravitational, elastic, or electrical—the steps to calculate potential energy involve identifying specific variables. Below is a step-by-step guide for calculating potential energy for different systems.
Gravitational Potential Energy
Gravitational potential energy depends on the mass of an object, the height above a reference point, and the gravitational constant. Here’s how to calculate it:
- Identify the mass (m): Determine the mass of the object in kilograms (kg).
- Measure the height (h): Identify the height of the object relative to a chosen reference point, such as the ground, in meters (m).
- Use the gravitational constant (g): On Earth, the gravitational constant is approximately 9.8 m/s².
- Apply the formula: The formula for gravitational potential energy is: U = m × g × h
Example Calculation:
Suppose you have a 5 kg object lifted to a height of 10 meters.
- Mass (m) = 5 kg
- Height (h) = 10 m
- Gravitational constant (g) = 9.8 m/s²
Now, calculate the potential energy:
PE = 5 kg × 9.8 m/s² × 10 m = 490 Joules
The gravitational potential energy is 490 Joules.
Elastic Potential Energy
Elastic potential energy is the energy stored in objects that can be stretched or compressed, such as springs. To calculate it, follow these steps:
- Determine the spring constant (k): The spring constant measures the stiffness of the spring in Newtons per meter (N/m).
- Measure the displacement (x): Identify how much the spring is stretched or compressed from its equilibrium position, in meters (m).
- Apply the formula: The formula for elastic potential energy is: U = 1/2 × k × x²
Example Calculation:
Assume you compress a spring with a spring constant of 300 N/m by 0.2 meters.
- Spring constant (k) = 300 N/m
- Displacement (x) = 0.2 m
Now, calculate the potential energy:
U = 1/2 × 300 N/m × (0.2 m)² = 6 Joules
The elastic potential energy is 6 Joules.
Electrical Potential Energy
Electrical potential energy arises from the interaction between charged particles. To calculate it, you’ll need to know the charges and the distance between them.
- Identify the charges (q₁ and q₂): Measure the magnitudes of the two charges in Coulombs (C).
- Measure the distance (r): Determine the distance between the charges in meters (m).
- Use Coulomb’s constant (kₑ): Coulomb’s constant is approximately 8.99 × 10⁹ N·m²/C².
- Apply the formula: The formula for electrical potential energy is: U = kₑ × (q₁ × q₂) / r
Example Calculation:
Suppose you have two charges of 2 × 10⁻⁶ C and 3 × 10⁻⁶ C separated by a distance of 0.05 meters.
- Charge q₁ = 2 × 10⁻⁶ C
- Charge q₂ = 3 × 10⁻⁶ C
- Distance (r) = 0.05 m
- Coulomb’s constant (kₑ) = 8.99 × 10⁹ N·m²/C²
Now, calculate the potential energy:
U = (8.99 × 10⁹ N·m²/C²) × (2 × 10⁻⁶ C × 3 × 10⁻⁶ C) / 0.05 m = 1.08 Joules
The electrical potential energy is 1.08 Joules.
By following these steps, you can calculate potential energy for various systems. Whether you’re dealing with an object in a gravitational field, a compressed spring, or charged particles, understanding the variables and applying the appropriate formulas will help you determine the amount of stored energy in any system.
Potential Energy vs Kinetic Energy
Potential energy and kinetic energy are two forms of energy that play a vital role in physical systems. While potential energy is stored energy due to an object's position or configuration, kinetic energy is the energy an object possesses due to its motion. These energies are deeply interconnected, especially when considering energy conservation.
Kinetic Energy
Kinetic energy is the energy of motion, given by the formula:
KE = 1/2 × m × v²
Where:
- m = mass of the object (kg)
- v = velocity of the object (m/s)
Relationship Between Potential and Kinetic Energy
In many physical systems, potential energy is converted into kinetic energy and vice versa. For example, when an object falls, its gravitational potential energy decreases while its kinetic energy increases as it gains speed.
In a pendulum, the energy alternates between potential energy (at the highest point) and kinetic energy (at the lowest point). This relationship illustrates the principle of conservation of energy, which states that energy cannot be created or destroyed, only transformed.
Comparison Table
| Aspect | Potential Energy | Kinetic Energy |
|---|---|---|
| Definition | Energy stored due to position or configuration | Energy due to motion |
| Formula | U = m × g × h (Gravitational) | KE = 1/2 × m × v² |
| Type | Stored energy | Active energy |
| Example | A ball held at a height | A ball rolling downhill |
| Conversion | Converts to kinetic energy when released | Converts to potential energy when stopped |
Understanding both types of energy is crucial for analyzing physical systems, as it allows engineers and scientists to predict how energy will move and change form in various processes.

Real-World Examples of Potential Energy Calculations
Potential energy plays a crucial role in many real-world applications, from simple daily tasks to large-scale engineering projects. Below are detailed examples that walk through potential energy calculations.
Example 1: Potential Energy of a Book on a Shelf
Imagine placing a 2 kg book on a shelf 1.5 meters above the ground. To calculate the gravitational potential energy (U) stored in the book, use the formula:
U = m × g × h
Where:
- m = 2 kg (mass of the book)
- g = 9.8 m/s² (gravitational constant)
- h = 1.5 m (height above the ground)
Now, calculate the potential energy:
PE = 2 kg × 9.8 m/s² × 1.5 m = 29.4 Joules
Assumptions: We assume the gravitational constant remains uniform, and the height is measured relative to the ground as the reference point.
Example 2: Potential Energy of a Roller Coaster at the Top of a Hill
A roller coaster car with a mass of 500 kg is at the top of a hill 50 meters above the ground. The gravitational potential energy at the top of the hill is given by:
U = m × g × h
Where:
- m = 500 kg (mass of the roller coaster car)
- g = 9.8 m/s² (gravitational constant)
- h = 50 m (height of the hill)
Now, calculate the potential energy:
U = 500 kg × 9.8 m/s² × 50 m = 245,000 Joules
This stored potential energy will be converted to kinetic energy as the car descends.
Assumptions: We assume no energy loss due to friction or air resistance and that the entire mass of the car is concentrated at a single point.
Example 3: Potential Energy of Water Stored in a Dam
Consider water stored behind a dam at a height of 100 meters. If the mass of water is 1,000,000 kg, the gravitational potential energy can be calculated as:
U = m × g × h
Where:
- m = 1,000,000 kg (mass of water)
- g = 9.8 m/s² (gravitational constant)
- h = 100 m (height of the water)
Now, calculate the potential energy:
U = 1,000,000 kg × 9.8 m/s² × 100 m = 980,000,000 Joules
This energy can be harnessed to generate electricity as the water flows down.
Assumptions: We assume all water is at the same height and that there is no energy loss during the process.
These examples highlight the importance of potential energy in various scenarios, from everyday objects like books to large-scale systems like dams. By understanding how to calculate potential energy, you can predict how energy will be stored and released in different situations, helping to design more efficient systems.
Practical Applications in Engineering and Technology
Potential energy concepts are essential in various engineering fields, where energy storage and conversion play a critical role. From designing safe structures to innovating energy storage solutions, the practical applications of potential energy are vast and transformative.
- Structural engineering: Potential energy principles are applied in the design of buildings, bridges, and other structures to ensure they can withstand loads and forces. Engineers calculate the gravitational potential energy stored in structures to ensure stability during events like earthquakes or wind loads.
- Mechanical systems: Cranes, elevators, and hoists rely on potential energy to lift and lower loads. The energy stored when lifting a load is later converted to kinetic energy as the load descends, allowing for efficient operation.
- Energy storage: Batteries and pumped hydro storage systems use potential energy principles to store energy. In pumped hydro storage, water is pumped to a higher elevation during off-peak hours and released during peak demand to generate electricity through the conversion of stored potential energy to kinetic energy.
- Renewable energy: Innovative technologies like gravitational energy storage systems use potential energy to store renewable energy. These systems lift heavy masses during periods of excess electricity generation and release them to generate electricity when needed, creating a sustainable energy cycle.
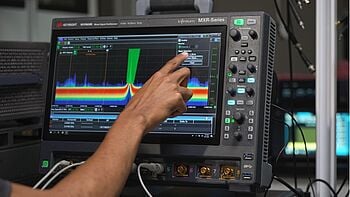
Tools for Measuring Potential Energy
Measuring potential energy accurately is essential in physics experiments and engineering projects, where precision can impact the outcome of a design or system.
Various tools and instruments are used to measure and analyze potential energy and related variables such as voltage, current, and displacement.
Here's an overview of the most commonly used tools, including how to use them effectively.
Oscilloscopes
Oscilloscopes are critical tools for visualizing energy-related signals and waveforms, such as voltage over time. They help engineers and physicists analyze the changes in potential energy by showing real-time data in graphical form.
Using an Oscilloscope
To use an oscilloscope, connect the probes to the circuit or system you’re analyzing. The oscilloscope will display a waveform that corresponds to the energy changes, such as voltage variations. You can adjust settings like the time base (horizontal scale) and voltage scale (vertical scale) to focus on the relevant parts of the waveform.
- Setup: Connect probes, set the time/div and volts/div dials, adjust trigger levels for stable waveforms, and ensure proper grounding.
- Data interpretation: Use the displayed waveform to analyze peaks, frequencies, and intervals where energy changes are most significant.
Other Relevant Tools
- Signal Generators:
- Used to create electrical signals, these are essential for testing systems that store or convert potential energy. Signal generators provide a controlled environment to analyze how systems respond to various inputs.
- Digital Multimeters (DMMs):
- DMMs measure voltage, current, and resistance, providing insight into the electrical aspects of potential energy in systems like capacitors or circuits. They help quantify the electrical potential energy stored in a system.
- Network Analyzers:
- Network Analyzers analyze the network parameters (such as impedance and signal transmission) of systems that convert or store energy, such as filters or power transmission lines.
Practical Tips for Tool Selection and Use
- Oscilloscopes: Choose an oscilloscope with a bandwidth suitable for your application. Ensure it has sufficient resolution and sampling rate to capture rapid energy fluctuations.
- Signal Generators: Select a generator that can output signals in the frequency and amplitude range required for your specific system.
- Digital Multimeters: Look for a DMM with high accuracy and low input impedance for precise measurements of energy-related variables.
- Network Analyzers: Ensure the analyzer has sufficient dynamic range and frequency coverage for characterizing energy storage systems in detail.
Comparison Table
| Tool | Application | Features | Best For |
|---|---|---|---|
| Oscilloscope | Visualizing waveforms of energy changes | Real-time display of signals, adjustable scales | Analyzing potential energy in dynamic systems |
| Signal Generator | Producing controlled electrical signals | Customizable frequency, amplitude | Testing and simulating energy storage devices |
| Digital Multimeter | Measuring voltage, current, resistance | High accuracy, multiple measurement modes | Quantifying potential energy in circuits |
| Network Analyzer | Analyzing network parameters of energy systems | Detailed signal transmission and impedance analysis | Characterizing energy conversion systems |
Choosing Premium Used Equipment
Investing in premium used equipment can save costs while still providing high-quality measurements. Reputable suppliers often offer calibrated, pre-owned devices that perform just as well as new ones, with quicker shipping times and reduced lead times.
Calibration is essential to ensure the accuracy and reliability of the equipment, especially when dealing with precise measurements like potential energy. Always prioritize calibrated equipment to maintain consistency and precision in your experiments and engineering projects.
By carefully selecting and properly using these tools, you can enhance the accuracy and reliability of your measurements, leading to more efficient and successful projects.
“For all used equipment, I offer my clients calibration and 1 y warranty.” – Keysight Account Manage
Ensure Accuracy with Keysight's Calibration Services
Accurately calculating potential energy is crucial for engineers and scientists working with mechanical, electrical, or structural systems. However, even with the correct formulas, inconsistent or imprecise measurements can lead to costly errors in your project. Tools like oscilloscopes, digital multimeters, and network analyzers are essential for these calculations, but only when they are properly calibrated.
At Keysight, we provide calibration services that ensure your instruments deliver precise, repeatable measurements. Whether you're measuring potential energy in electrical systems or analyzing dynamic mechanical forces, our calibrated equipment helps you maintain the accuracy you need.
With our solutions, you don’t have to worry about unreliable data disrupting your projects—our premium calibrated tools are designed to meet your demands.
We make reliable test results accessible and affordable, so you can focus on innovation and solving complex engineering challenges with confidence. With Keysight's Premium Used Equipment, you can trust that your equipment will deliver accurate data every time.
Whenever You’re Ready, Here Are 5 Ways We Can Help You
- Browse our premium used network analyzers, oscilloscopes, signal analyzers and waveform generators.
- Call tech support US: 1 800 829-4444
Press #, then 2. Hours: 7am – 5pm MT, Mon– Fri - Talk to our sales support team by clicking the icon (bottom right corner) on every offer page
- Create an account to get price alerts and access to exclusive waitlists
- Talk to your account manager about your specific needs












































